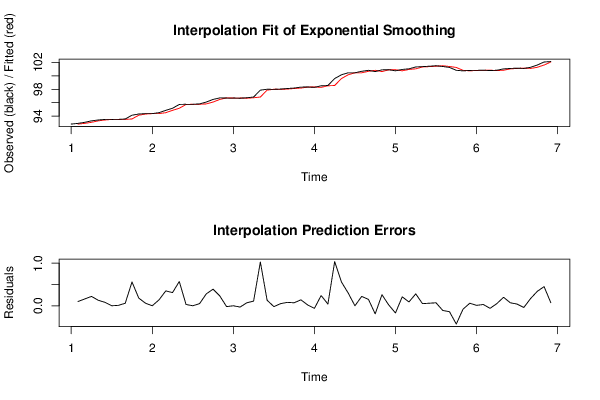
Estimated Parameters of Exponential Smoothing
Parameter	Value
alpha	0.999919206385201
beta	FALSE
gamma	FALSE

Interpolation Forecasts of Exponential Smoothing
t	Observed	Fitted	Residuals
2	92.9	92.8	0.100000000000009
3	93.06	92.8999919206385	0.160008079361475
4	93.28	93.0599870723689	0.220012927631132
5	93.41	93.2799822243603	0.130017775639715
6	93.49	93.4099894953939	0.0800105046060935
7	93.49	93.4899935356621	6.46433788631384e-06
8	93.5	93.4899999994777	0.0100000005222824
9	93.56	93.4999991920638	0.0600008079361913
10	94.12	93.5599951523178	0.560004847682166
11	94.3	94.1199547551841	0.180045244815943
12	94.36	94.2999854534938	0.060014546506153
13	94.36	94.3599951512078	4.84879215889578e-06
14	94.5	94.3599999996082	0.140000000391751
15	94.85	94.4999886888939	0.350011311106101
16	95.16	94.849971721321	0.310028278679042
17	95.73	95.1599749516947	0.57002504830534
18	95.76	95.7299539456158	0.0300460543841865
19	95.76	95.7599975724707	2.42752933843349e-06
20	95.81	95.7599999998039	0.0500000001961212
21	96.09	95.8099959603192	0.280004039680762
22	96.48	96.0899773774615	0.390022622538524
23	96.71	96.4799684886625	0.230031511337515
24	96.69	96.7099814149227	-0.0199814149226683
25	96.69	96.6900016143707	-1.61437073131765e-06
26	96.66	96.6900000001304	-0.0300000001304284
27	96.73	96.6600024238085	0.0699975761915539
28	96.84	96.7299943446428	0.110005655357213
29	97.87	96.8399911122455	1.03000888775455
30	98	97.8699167818587	0.130083218141323
31	97.98	97.9999894901066	-0.0199894901065818
32	98.03	97.9800016150232	0.0499983849768313
33	98.11	98.0299959604498	0.0800040395502464
34	98.18	98.1099935361844	0.0700064638155595
35	98.32	98.1799943439247	0.14000565607526
36	98.34	98.3199886884369	0.0200113115630671
37	98.28	98.3399983832138	-0.0599983832137951
38	98.52	98.2800048474863	0.239995152513728
39	98.56	98.5199806099241	0.040019390075912
40	99.6	98.5599967666888	1.04000323331118
41	100.16	99.5999159743794	0.560084025620625
42	100.46	100.159954748787	0.300045251213021
43	100.46	100.45997575826	2.42417404479056e-05
44	100.68	100.459999998041	0.220000001958596
45	100.83	100.679982225405	0.150017774595398
46	100.64	100.829987879522	-0.189987879521709
47	100.9	100.640015349808	0.25998465019245
48	100.92	100.8999789949	0.0200210050996787
49	100.75	100.919998382431	-0.169998382430634
50	100.96	100.750013734784	0.209986265216159
51	101.05	100.959983034451	0.0900169655494381
52	101.33	101.049992727204	0.280007272796041
53	101.38	101.3299773772	0.0500226227997445
54	101.44	101.379995958491	0.0600040415085203
55	101.51	101.439995152057	0.070004847943423
56	101.4	101.509994344055	-0.109994344055281
57	101.26	101.400008886841	-0.140008886840675
58	100.83	101.260011311824	-0.430011311824074
59	100.75	100.830034742168	-0.0800347421682801
60	100.81	100.750006466296	0.0599935337038744
61	100.82	100.809995152906	0.01000484709445
62	100.85	100.819999191672	0.0300008083277561
63	100.79	100.849997576126	-0.0599975761262357
64	100.84	100.790004847421	0.0499951525789442
65	101.04	100.839995960711	0.200004039289098
66	101.11	101.039983840951	0.0700161590493025
67	101.15	101.109994343141	0.0400056568585825
68	101.11	101.149996767798	-0.039996767798371
69	101.28	101.110003231483	0.169996768516555
70	101.62	101.279986265347	0.340013734653439
71	102.07	101.619972529061	0.450027470938693
72	102.14	102.069963640654	0.0700363593461475

Extrapolation Forecasts of Exponential Smoothing
t	Forecast	95% Lower Bound	95% Upper Bound
73	102.139994341509	101.686987159418	102.593001523601
74	102.139994341509	101.499371320391	102.780617362628
75	102.139994341509	101.355405147505	102.924583535513
76	102.139994341509	101.234034876904	103.045953806115
77	102.139994341509	101.127104959756	103.152883723263
78	102.139994341509	101.030432604683	103.249556078336
79	102.139994341509	100.941532996288	103.338455686731
80	102.139994341509	100.858787120116	103.421201562903
81	102.139994341509	100.781070395031	103.498918287987
82	102.139994341509	100.707564014941	103.572424668078
83	102.139994341509	100.637649844188	103.642338838831
84	102.139994341509	100.570847650854	103.709141032164