Blog & Share Statistical Computations at FreeStatistics.org

Home » date » 2009 » Nov » 20 »

*The author of this computation has been verified*

R Software Module: /rwasp_multipleregression.wasp (opens new window with default values)

Title produced by software: Multiple Regression

Date of computation: Fri, 20 Nov 2009 11:48:57 -0700

Cite this page as follows:

Statistical Computations at FreeStatistics.org, Office for Research Development and Education, URL http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p.htm/, Retrieved Fri, 20 Nov 2009 19:49:58 +0100

BibTeX entries for LaTeX users:

@Manual{KEY,
    author = {{YOUR NAME}},
    publisher = {Office for Research Development and Education},
    title = {Statistical Computations at FreeStatistics.org, URL http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p.htm/},
    year = {2009},
}
@Manual{R,
    title = {R: A Language and Environment for Statistical Computing},
    author = {{R Development Core Team}},
    organization = {R Foundation for Statistical Computing},
    address = {Vienna, Austria},
    year = {2009},
    note = {{ISBN} 3-900051-07-0},
    url = {http://www.R-project.org},
}

Original text written by user:

IsPrivate?

No (this computation is public)

User-defined keywords:

Dataseries X:

» Textbox « » Textfile « » CSV «

2187 18.8 1855 2218 1852 18.2 2187 1855 1570 18 1852 2187 1851 19 1570 1852 1954 20.7 1851 1570 1828 21.2 1954 1851 2251 20.7 1828 1954 2277 19.6 2251 1828 2085 18.6 2277 2251 2282 18.7 2085 2277 2266 23.8 2282 2085 1878 24.9 2266 2282 2267 24.8 1878 2266 2069 23.8 2267 1878 1746 22.3 2069 2267 2299 21.7 1746 2069 2360 20.7 2299 1746 2214 19.7 2360 2299 2825 18.4 2214 2360 2355 17.4 2825 2214 2333 17 2355 2825 3016 18 2333 2355 2155 23.8 3016 2333 2172 25.5 2155 3016 2150 25.6 2172 2155 2533 23.7 2150 2172 2058 22 2533 2150 2160 21.3 2058 2533 2260 20.7 2160 2058 2498 20.4 2260 2160 2695 20.3 2498 2260 2799 20.4 2695 2498 2946 19.8 2799 2695 2930 19.5 2946 2799 2318 23.1 2930 2946 2540 23.5 2318 2930 2570 23.5 2540 2318 2669 22.9 2570 2540 2450 21.9 2669 2570 2842 21.5 2450 2669 3440 20.5 2842 2450 2678 20.2 3440 2842 2981 19.4 2678 3440 2260 19.2 2981 2678 2844 18.8 2260 2981 2546 18.8 2844 2260 2456 22.6 2546 2844 2295 23.3 2456 2546 2379 23 2295 2456 2479 21 etc...

Output produced by software:

Enter (or paste) a matrix (table) containing all data (time) series. Every column represents a different variable and must be delimited by a space or Tab. Every row represents a period in time (or category) and must be delimited by hard returns. The easiest way to enter data is to copy and paste a block of spreadsheet cells. Please, do not use commas or spaces to seperate groups of digits!

Summary of computational transaction
Raw Input	view raw input (R code)
Raw Output	view raw output of R engine
Computing time	5 seconds
R Server	'Gwilym Jenkins' @ 72.249.127.135

Multiple Linear Regression - Estimated Regression Equation

Y[t] = -354.405794860257 + 29.7764909584763X[t] + 0.290728283519998Y1[t] + 0.417952030129352Y2[t] + 349.124779045002M1[t] + 399.919848770249M2[t] + 18.1139165012463M3[t] + 433.072144216138M4[t] + 677.964093127146M5[t] + 325.673309002917M6[t] + 672.482518326726M7[t] + 384.104420017886M8[t] + 406.540579208735M9[t] + 707.44158962931M10[t] + 52.8413553507744M11[t] + 1.93405432247590t + e[t]

Multiple Linear Regression - Ordinary Least Squares
Variable	Parameter	S.D.	T-STAT H0: parameter = 0	2-tail p-value	1-tail p-value
(Intercept)	-354.405794860257	656.464213	-0.5399	0.592137	0.296069
X	29.7764909584763	23.707188	1.256	0.216054	0.108027
Y1	0.290728283519998	0.140916	2.0631	0.045317	0.022659
Y2	0.417952030129352	0.145559	2.8714	0.006379	0.003189
M1	349.124779045002	165.378287	2.1111	0.04076	0.02038
M2	399.919848770249	184.340475	2.1695	0.035756	0.017878
M3	18.1139165012463	187.011243	0.0969	0.923298	0.461649
M4	433.072144216138	185.205537	2.3383	0.024206	0.012103
M5	677.964093127146	213.776534	3.1714	0.002833	0.001417
M6	325.673309002917	205.220172	1.5869	0.120026	0.060013
M7	672.482518326726	197.407667	3.4066	0.00146	0.00073
M8	384.104420017886	224.188549	1.7133	0.094029	0.047015
M9	406.540579208735	207.312869	1.961	0.056532	0.028266
M10	707.44158962931	218.701478	3.2347	0.002375	0.001188
M11	52.8413553507744	180.631129	0.2925	0.771316	0.385658
t	1.93405432247590	2.32745	0.831	0.410687	0.205343

Multiple Linear Regression - Regression Statistics
Multiple R	0.824472354264483
R-squared	0.679754662946419
Adjusted R-squared	0.565381328284426
F-TEST (value)	5.94329670421251
F-TEST (DF numerator)	15
F-TEST (DF denominator)	42
p-value	2.32630427787761e-06
Multiple Linear Regression - Residual Statistics
Residual Standard Deviation	231.92820960305
Sum Squared Residuals	2259209.16520640

Multiple Linear Regression - Actuals, Interpolation, and Residuals
Time or Index	Actuals	Interpolation Forecast	Residuals Prediction Error
1	2187	2022.76963728308	164.230362716923
2	1852	2002.43806994740	-150.438069947396
3	1570	1657.97699283292	-87.9769928329201
4	1851	1882.64645978279	-31.6464597827909
5	1954	2143.92467281833	-189.924672818327
6	1828	1955.84572216472	-127.845722164720
7	2251	2296.11803571157	-45.1180357115703
8	2277	2047.23595980354	229.764040196457
9	2085	2226.18232647463	-141.182326474627
10	2282	2487.04196266105	-205.041962661050
11	2266	1963.26256866182	302.737431338177
12	1878	2022.79430508701	-144.794305087011
13	2267	2251.38568287081	15.6143171291875
14	2069	2225.26623055915	-156.266230559150
15	1746	1905.74875575827	-159.748755758267
16	2299	2128.11540567798	170.884594322023
17	2360	2370.93915300776	-10.9391530077634
18	2214	2239.66783020379	-25.6678302037859
19	2825	2532.75040004802	292.249599951978
20	2355	2333.14384993501	21.8561500649853
21	2333	2464.32986421958	-131.329864219584
22	3016	2594.10794352288	421.892056477123
23	2155	2303.51788410729	-148.517884107292
24	2172	2338.37480217603	-166.374802176033
25	2150	2337.49696751783	-187.496967517826
26	2533	2334.35992101920	198.640078980798
27	2058	2006.02199636858	51.9780036314203
28	2160	2424.05042760256	-264.050427602557
29	2260	2484.13760686855	-224.137606868552
30	2498	2196.55186520445	301.448134795550
31	2695	2653.30601424558	41.6939857544177
32	2799	2526.58567437929	272.414325620709
33	2946	2645.66228473909	300.337715260908
34	2930	3025.76847100549	-95.7684710054936
35	2318	2537.08495439264	-219.084954392643
36	2540	2313.47530775143	226.524692248574
37	2570	2473.28917762118	96.7108223788196
38	2669	2609.65960628813	59.3403937118668
39	2450	2241.33189835549	208.668101644509
40	2842	2624.02134090139	217.978659098606
41	3440	2863.50484571791	576.495154282087
42	2678	2841.90787798428	-163.907877984282
43	2981	3195.2303108389	-214.230310838900
44	2260	2672.44219160883	-412.442191608833
45	2844	2601.92618145004	242.073818549958
46	2546	2773.20315004551	-227.203150045510
47	2456	2391.13459283824	64.865407161758
48	2295	2210.35558498553	84.6444150144698
49	2379	2468.05853470710	-89.0585347071042
50	2479	2430.27617218612	48.7238278138816
51	2057	2069.92035668474	-12.9203566847428
52	2280	2373.16636603528	-93.1663660352822
53	2351	2502.49372158744	-151.493721587444
54	2276	2260.02670444276	15.9732955572386
55	2548	2622.59523915593	-74.5952391559254
56	2311	2422.59232427332	-111.592324273318
57	2201	2470.89934311665	-269.899343116654
58	2725	2618.87847276507	106.121527234931

Goldfeld-Quandt test for Heteroskedasticity
p-values	Alternative Hypothesis
breakpoint index	greater	2-sided	less
19	0.0288054233547557	0.0576108467095114	0.971194576645244
20	0.0259396296345399	0.0518792592690798	0.97406037036546
21	0.049423175173336	0.098846350346672	0.950576824826664
22	0.024667628869918	0.049335257739836	0.975332371130082
23	0.0126697804682836	0.0253395609365672	0.987330219531716
24	0.00753926751212804	0.0150785350242561	0.992460732487872
25	0.176084655598167	0.352169311196333	0.823915344401833
26	0.108074653596999	0.216149307193997	0.891925346403001
27	0.0670796891691306	0.134159378338261	0.93292031083087
28	0.0872551949980472	0.174510389996094	0.912744805001953
29	0.429210506070552	0.858421012141104	0.570789493929448
30	0.368034566840438	0.736069133680875	0.631965433159562
31	0.349266744511663	0.698533489023326	0.650733255488337
32	0.271655285113476	0.543310570226952	0.728344714886524
33	0.380826456613756	0.761652913227511	0.619173543386244
34	0.285140417896361	0.570280835792722	0.714859582103639
35	0.27662058223366	0.55324116446732	0.72337941776634
36	0.192462744525136	0.384925489050272	0.807537255474864
37	0.123598085817568	0.247196171635136	0.876401914182432
38	0.103621127666641	0.207242255333281	0.89637887233336
39	0.0915090611975366	0.183018122395073	0.908490938802463

Meta Analysis of Goldfeld-Quandt test for Heteroskedasticity
Description	# significant tests	% significant tests	OK/NOK
1% type I error level	0	0	OK
5% type I error level	3	0.142857142857143	NOK
10% type I error level	6	0.285714285714286	NOK

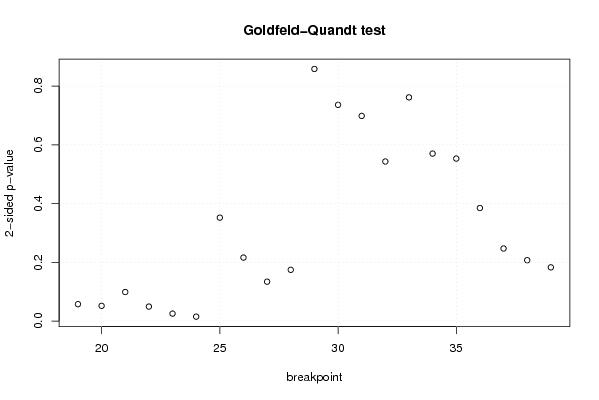
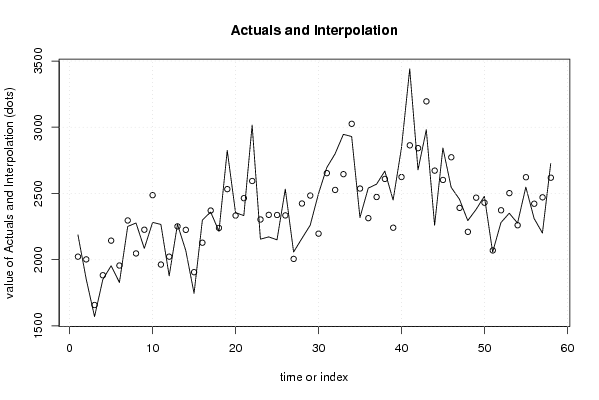
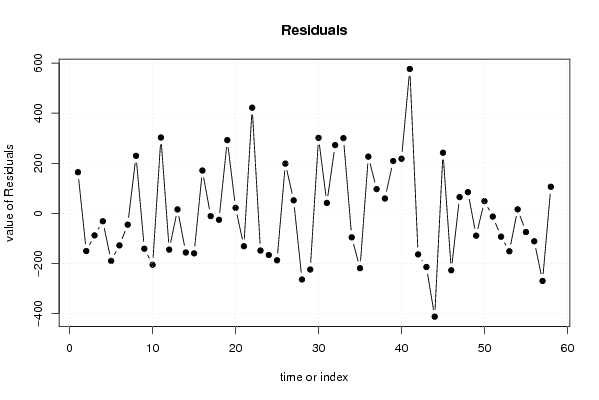
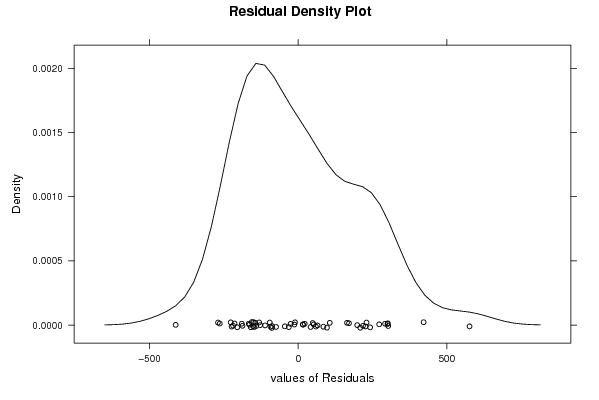
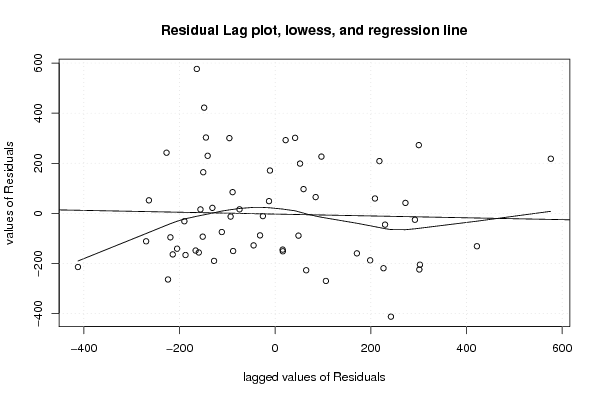
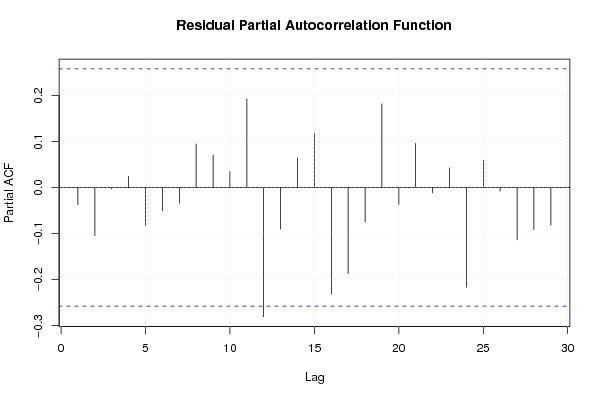
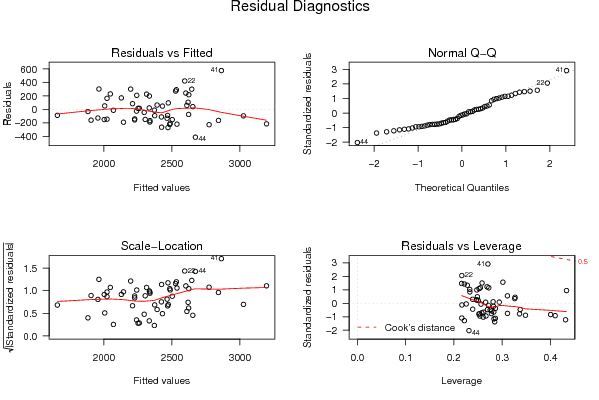
Charts produced by software:

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/10lovj1258742931.png (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/10lovj1258742931.ps (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/1as2j1258742931.png (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/1as2j1258742931.ps (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/20z4k1258742931.png (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/20z4k1258742931.ps (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/3gbzh1258742931.png (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/3gbzh1258742931.ps (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/4syx01258742931.png (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/4syx01258742931.ps (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/5xq3j1258742931.png (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/5xq3j1258742931.ps (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/6kui41258742931.png (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/6kui41258742931.ps (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/7rmq61258742931.png (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/7rmq61258742931.ps (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/8qg951258742931.png (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/8qg951258742931.ps (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/94fhe1258742931.png (open in new window)

http://www.freestatistics.org/blog/date/2009/Nov/20/t1258742986pf51h0ewn1l2v5p/94fhe1258742931.ps (open in new window)

Parameters (Session):

par1 = 1 ; par2 = Include Monthly Dummies ; par3 = Linear Trend ;

Parameters (R input):

par1 = 1 ; par2 = Include Monthly Dummies ; par3 = Linear Trend ;

R code (references can be found in the software module):

library(lattice)

library(lmtest)

n25 <- 25 #minimum number of obs. for Goldfeld-Quandt test

par1 <- as.numeric(par1)

x <- t(y)

k <- length(x[1,])

n <- length(x[,1])

x1 <- cbind(x[,par1], x[,1:k!=par1])

mycolnames <- c(colnames(x)[par1], colnames(x)[1:k!=par1])

colnames(x1) <- mycolnames #colnames(x)[par1]

x <- x1

if (par3 == 'First Differences'){

x2 <- array(0, dim=c(n-1,k), dimnames=list(1:(n-1), paste('(1-B)',colnames(x),sep='')))

for (i in 1:n-1) {

for (j in 1:k) {

x2[i,j] <- x[i+1,j] - x[i,j]

}

}

x <- x2

}

if (par2 == 'Include Monthly Dummies'){

x2 <- array(0, dim=c(n,11), dimnames=list(1:n, paste('M', seq(1:11), sep ='')))

for (i in 1:11){

x2[seq(i,n,12),i] <- 1

}

x <- cbind(x, x2)

}

if (par2 == 'Include Quarterly Dummies'){

x2 <- array(0, dim=c(n,3), dimnames=list(1:n, paste('Q', seq(1:3), sep ='')))

for (i in 1:3){

x2[seq(i,n,4),i] <- 1

}

x <- cbind(x, x2)

}

k <- length(x[1,])

if (par3 == 'Linear Trend'){

x <- cbind(x, c(1:n))

colnames(x)[k+1] <- 't'

}

x

k <- length(x[1,])

df <- as.data.frame(x)

(mylm <- lm(df))

(mysum <- summary(mylm))

if (n > n25) {

kp3 <- k + 3

nmkm3 <- n - k - 3

gqarr <- array(NA, dim=c(nmkm3-kp3+1,3))

numgqtests <- 0

numsignificant1 <- 0

numsignificant5 <- 0

numsignificant10 <- 0

for (mypoint in kp3:nmkm3) {

j <- 0

numgqtests <- numgqtests + 1

for (myalt in c('greater', 'two.sided', 'less')) {

j <- j + 1

gqarr[mypoint-kp3+1,j] <- gqtest(mylm, point=mypoint, alternative=myalt)$p.value

}

if (gqarr[mypoint-kp3+1,2] < 0.01) numsignificant1 <- numsignificant1 + 1

if (gqarr[mypoint-kp3+1,2] < 0.05) numsignificant5 <- numsignificant5 + 1

if (gqarr[mypoint-kp3+1,2] < 0.10) numsignificant10 <- numsignificant10 + 1

}

gqarr

}

bitmap(file='test0.png')

plot(x[,1], type='l', main='Actuals and Interpolation', ylab='value of Actuals and Interpolation (dots)', xlab='time or index')

points(x[,1]-mysum$resid)

grid()

dev.off()

bitmap(file='test1.png')

plot(mysum$resid, type='b', pch=19, main='Residuals', ylab='value of Residuals', xlab='time or index')

grid()

dev.off()

bitmap(file='test2.png')

hist(mysum$resid, main='Residual Histogram', xlab='values of Residuals')

grid()

dev.off()

bitmap(file='test3.png')

densityplot(~mysum$resid,col='black',main='Residual Density Plot', xlab='values of Residuals')

dev.off()

bitmap(file='test4.png')

qqnorm(mysum$resid, main='Residual Normal Q-Q Plot')

qqline(mysum$resid)

grid()

dev.off()

(myerror <- as.ts(mysum$resid))

bitmap(file='test5.png')

dum <- cbind(lag(myerror,k=1),myerror)

dum

dum1 <- dum[2:length(myerror),]

dum1

z <- as.data.frame(dum1)

z

plot(z,main=paste('Residual Lag plot, lowess, and regression line'), ylab='values of Residuals', xlab='lagged values of Residuals')

lines(lowess(z))

abline(lm(z))

grid()

dev.off()

bitmap(file='test6.png')

acf(mysum$resid, lag.max=length(mysum$resid)/2, main='Residual Autocorrelation Function')

grid()

dev.off()

bitmap(file='test7.png')

pacf(mysum$resid, lag.max=length(mysum$resid)/2, main='Residual Partial Autocorrelation Function')

grid()

dev.off()

bitmap(file='test8.png')

opar <- par(mfrow = c(2,2), oma = c(0, 0, 1.1, 0))

plot(mylm, las = 1, sub='Residual Diagnostics')

par(opar)

dev.off()

if (n > n25) {

bitmap(file='test9.png')

plot(kp3:nmkm3,gqarr[,2], main='Goldfeld-Quandt test',ylab='2-sided p-value',xlab='breakpoint')

grid()

dev.off()

}

load(file='createtable')

a<-table.start()

a<-table.row.start(a)

a<-table.element(a, 'Multiple Linear Regression - Estimated Regression Equation', 1, TRUE)

a<-table.row.end(a)

myeq <- colnames(x)[1]

myeq <- paste(myeq, '[t] = ', sep='')

for (i in 1:k){

if (mysum$coefficients[i,1] > 0) myeq <- paste(myeq, '+', '')

myeq <- paste(myeq, mysum$coefficients[i,1], sep=' ')

if (rownames(mysum$coefficients)[i] != '(Intercept)') {

myeq <- paste(myeq, rownames(mysum$coefficients)[i], sep='')

if (rownames(mysum$coefficients)[i] != 't') myeq <- paste(myeq, '[t]', sep='')

}

}

myeq <- paste(myeq, ' + e[t]')

a<-table.row.start(a)

a<-table.element(a, myeq)

a<-table.row.end(a)

a<-table.end(a)

table.save(a,file='mytable1.tab')

a<-table.start()

a<-table.row.start(a)

a<-table.element(a,hyperlink('http://www.xycoon.com/ols1.htm','Multiple Linear Regression - Ordinary Least Squares',''), 6, TRUE)

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a,'Variable',header=TRUE)

a<-table.element(a,'Parameter',header=TRUE)

a<-table.element(a,'S.D.',header=TRUE)

a<-table.element(a,'T-STAT<br />H0: parameter = 0',header=TRUE)

a<-table.element(a,'2-tail p-value',header=TRUE)

a<-table.element(a,'1-tail p-value',header=TRUE)

a<-table.row.end(a)

for (i in 1:k){

a<-table.row.start(a)

a<-table.element(a,rownames(mysum$coefficients)[i],header=TRUE)

a<-table.element(a,mysum$coefficients[i,1])

a<-table.element(a, round(mysum$coefficients[i,2],6))

a<-table.element(a, round(mysum$coefficients[i,3],4))

a<-table.element(a, round(mysum$coefficients[i,4],6))

a<-table.element(a, round(mysum$coefficients[i,4]/2,6))

a<-table.row.end(a)

}

a<-table.end(a)

table.save(a,file='mytable2.tab')

a<-table.start()

a<-table.row.start(a)

a<-table.element(a, 'Multiple Linear Regression - Regression Statistics', 2, TRUE)

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a, 'Multiple R',1,TRUE)

a<-table.element(a, sqrt(mysum$r.squared))

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a, 'R-squared',1,TRUE)

a<-table.element(a, mysum$r.squared)

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a, 'Adjusted R-squared',1,TRUE)

a<-table.element(a, mysum$adj.r.squared)

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a, 'F-TEST (value)',1,TRUE)

a<-table.element(a, mysum$fstatistic[1])

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a, 'F-TEST (DF numerator)',1,TRUE)

a<-table.element(a, mysum$fstatistic[2])

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a, 'F-TEST (DF denominator)',1,TRUE)

a<-table.element(a, mysum$fstatistic[3])

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a, 'p-value',1,TRUE)

a<-table.element(a, 1-pf(mysum$fstatistic[1],mysum$fstatistic[2],mysum$fstatistic[3]))

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a, 'Multiple Linear Regression - Residual Statistics', 2, TRUE)

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a, 'Residual Standard Deviation',1,TRUE)

a<-table.element(a, mysum$sigma)

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a, 'Sum Squared Residuals',1,TRUE)

a<-table.element(a, sum(myerror*myerror))

a<-table.row.end(a)

a<-table.end(a)

table.save(a,file='mytable3.tab')

a<-table.start()

a<-table.row.start(a)

a<-table.element(a, 'Multiple Linear Regression - Actuals, Interpolation, and Residuals', 4, TRUE)

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a, 'Time or Index', 1, TRUE)

a<-table.element(a, 'Actuals', 1, TRUE)

a<-table.element(a, 'Interpolation<br />Forecast', 1, TRUE)

a<-table.element(a, 'Residuals<br />Prediction Error', 1, TRUE)

a<-table.row.end(a)

for (i in 1:n) {

a<-table.row.start(a)

a<-table.element(a,i, 1, TRUE)

a<-table.element(a,x[i])

a<-table.element(a,x[i]-mysum$resid[i])

a<-table.element(a,mysum$resid[i])

a<-table.row.end(a)

}

a<-table.end(a)

table.save(a,file='mytable4.tab')

if (n > n25) {

a<-table.start()

a<-table.row.start(a)

a<-table.element(a,'Goldfeld-Quandt test for Heteroskedasticity',4,TRUE)

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a,'p-values',header=TRUE)

a<-table.element(a,'Alternative Hypothesis',3,header=TRUE)

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a,'breakpoint index',header=TRUE)

a<-table.element(a,'greater',header=TRUE)

a<-table.element(a,'2-sided',header=TRUE)

a<-table.element(a,'less',header=TRUE)

a<-table.row.end(a)

for (mypoint in kp3:nmkm3) {

a<-table.row.start(a)

a<-table.element(a,mypoint,header=TRUE)

a<-table.element(a,gqarr[mypoint-kp3+1,1])

a<-table.element(a,gqarr[mypoint-kp3+1,2])

a<-table.element(a,gqarr[mypoint-kp3+1,3])

a<-table.row.end(a)

}

a<-table.end(a)

table.save(a,file='mytable5.tab')

a<-table.start()

a<-table.row.start(a)

a<-table.element(a,'Meta Analysis of Goldfeld-Quandt test for Heteroskedasticity',4,TRUE)

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a,'Description',header=TRUE)

a<-table.element(a,'# significant tests',header=TRUE)

a<-table.element(a,'% significant tests',header=TRUE)

a<-table.element(a,'OK/NOK',header=TRUE)

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a,'1% type I error level',header=TRUE)

a<-table.element(a,numsignificant1)

a<-table.element(a,numsignificant1/numgqtests)

if (numsignificant1/numgqtests < 0.01) dum <- 'OK' else dum <- 'NOK'

a<-table.element(a,dum)

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a,'5% type I error level',header=TRUE)

a<-table.element(a,numsignificant5)

a<-table.element(a,numsignificant5/numgqtests)

if (numsignificant5/numgqtests < 0.05) dum <- 'OK' else dum <- 'NOK'

a<-table.element(a,dum)

a<-table.row.end(a)

a<-table.row.start(a)

a<-table.element(a,'10% type I error level',header=TRUE)

a<-table.element(a,numsignificant10)

a<-table.element(a,numsignificant10/numgqtests)

if (numsignificant10/numgqtests < 0.1) dum <- 'OK' else dum <- 'NOK'

a<-table.element(a,dum)

a<-table.row.end(a)

a<-table.end(a)

table.save(a,file='mytable6.tab')

}

This work is licensed under a Creative Commons Attribution-Noncommercial-Share Alike 3.0 License.

Software written by Ed van Stee & Patrick Wessa

Disclaimer

Information provided on this web site is provided "AS IS" without warranty of any kind, either express or implied, including, without limitation, warranties of merchantability, fitness for a particular purpose, and noninfringement. We use reasonable efforts to include accurate and timely information and periodically update the information, and software without notice. However, we make no warranties or representations as to the accuracy or completeness of such information (or software), and we assume no liability or responsibility for errors or omissions in the content of this web site, or any software bugs in online applications. Your use of this web site is AT YOUR OWN RISK. Under no circumstances and under no legal theory shall we be liable to you or any other person for any direct, indirect, special, incidental, exemplary, or consequential damages arising from your access to, or use of, this web site.

We may request personal information to be submitted to our servers in order to be able to:

personalize online software applications according to your needs
enforce strict security rules with respect to the data that you upload (e.g. statistical data)
manage user sessions of online applications
alert you about important changes or upgrades in resources or applications

We NEVER allow other companies to directly offer registered users information about their products and services. Banner references and hyperlinks of third parties NEVER contain any personal data of the visitor.

We do NOT sell, nor transmit by any means, personal information, nor statistical data series uploaded by you to third parties.

We carefully protect your data from loss, misuse, alteration, and destruction. However, at any time, and under any circumstance you are solely responsible for managing your passwords, and keeping them secret.

We store a unique ANONYMOUS USER ID in the form of a small 'Cookie' on your computer. This allows us to track your progress when using this website which is necessary to create state-dependent features. The cookie is used for NO OTHER PURPOSE. At any time you may opt to disallow cookies from this website - this will not affect other features of this website.

We examine cookies that are used by third-parties (banner and online ads) very closely: abuse from third-parties automatically results in termination of the advertising contract without refund. We have very good reason to believe that the cookies that are produced by third parties (banner ads) do NOT cause any privacy or security risk.

FreeStatistics.org is safe. There is no need to download any software to use the applications and services contained in this website. Hence, your system's security is not compromised by their use, and your personal data - other than data you submit in the account application form, and the user-agent information that is transmitted by your browser - is never transmitted to our servers.

As a general rule, we do not log on-line behavior of individuals (other than normal logging of webserver 'hits'). However, in cases of abuse, hacking, unauthorized access, Denial of Service attacks, illegal copying, hotlinking, non-compliance with international webstandards (such as robots.txt), or any other harmful behavior, our system engineers are empowered to log, track, identify, publish, and ban misbehaving individuals - even if this leads to ban entire blocks of IP addresses, or disclosing user's identity.

FreeStatistics.org is powered by